Introduction to Fractions

• Fractions were first introduced in measurement problems, to express a quantity that is less than a whole unit. The word fraction comes from the Latin word “fractio” which means “the act of breaking into pieces”.
•  Fraction: A fraction is an ordered pair of integers a and b, with b not equal to 0, written . The integer a is called the numerator of the fraction, and the integer b is called the denominator of the fraction.

            3 Models for Fractions:  1.) Part to whole  2.) Divison  3.) Ratio

1.) Part to Whole-  To interpret the meaning of any fraction we must
agree on the unit (The "Whole") ex: a cup, an inch, the area of a hexagon, a whole pizza, etc. Understand that the unit is subdivided into b parts of equal size
understand that we are considering a parts of the unit.
            Area Models: are the shaded part of a shape

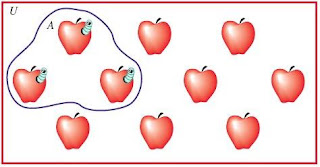
Set Model-  A finite set of objects U is chosen to represent the unit which is divided into n(U) = equal parts. A fraction is then visualized by circling the number of objects that represent the numerator of the fraction.
= 3/10
Number-Line Model: The unit is represented by the length of the line segment between 0 and 1 on the number line. That portion of the number line is subdivided into equally sized segments.

2.) Division- For any numbers A and B with b not equal to 0  For Example: to compute 3 divided by 4, we use the sharing concept of division

3.) Ratio 
-In this case, fractions are used to compare one amount to another.
-Using Cuisenaire Rods, we compare the lengths of two rods.
-For example, if we compare the dark green and red rods. How many red rods does it take to have the same length as the dark green rod? What fraction does the red rod represent if the dark green rod is considered the unit? How about comparing the red rod to the yellow?